Measurement 3 4 5 Triangle Angles

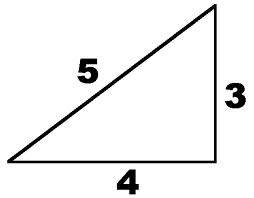
3 4 5 Triangle
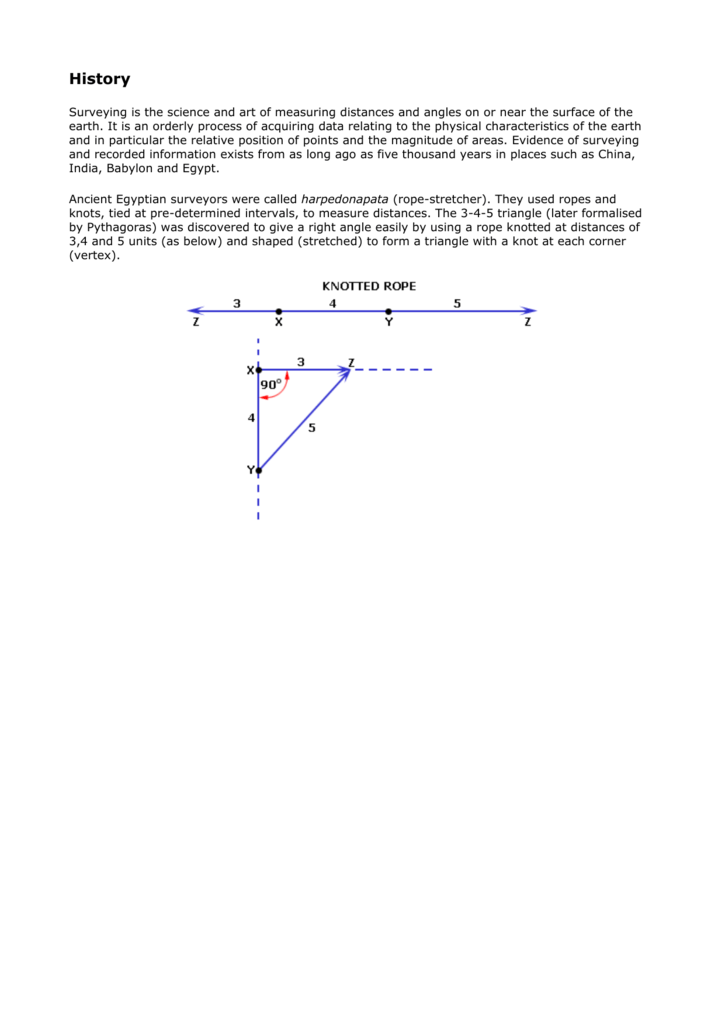
An Offset Survey

Construct Atriangle Having Perimeter 12 5 Cm And The Ratio Of Angles 3 4 5
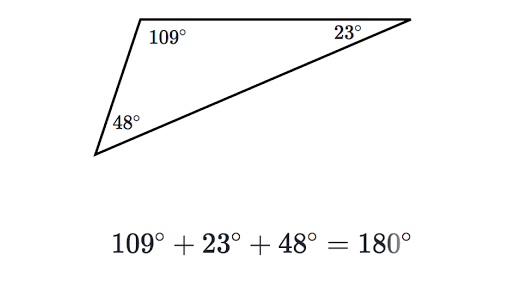
Angles Of A Triangle Review Geometry Article Khan Academy
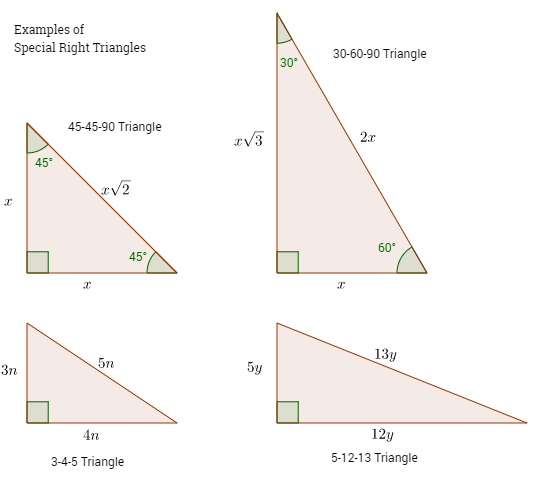
Special Right Triangles Solutions Examples Videos
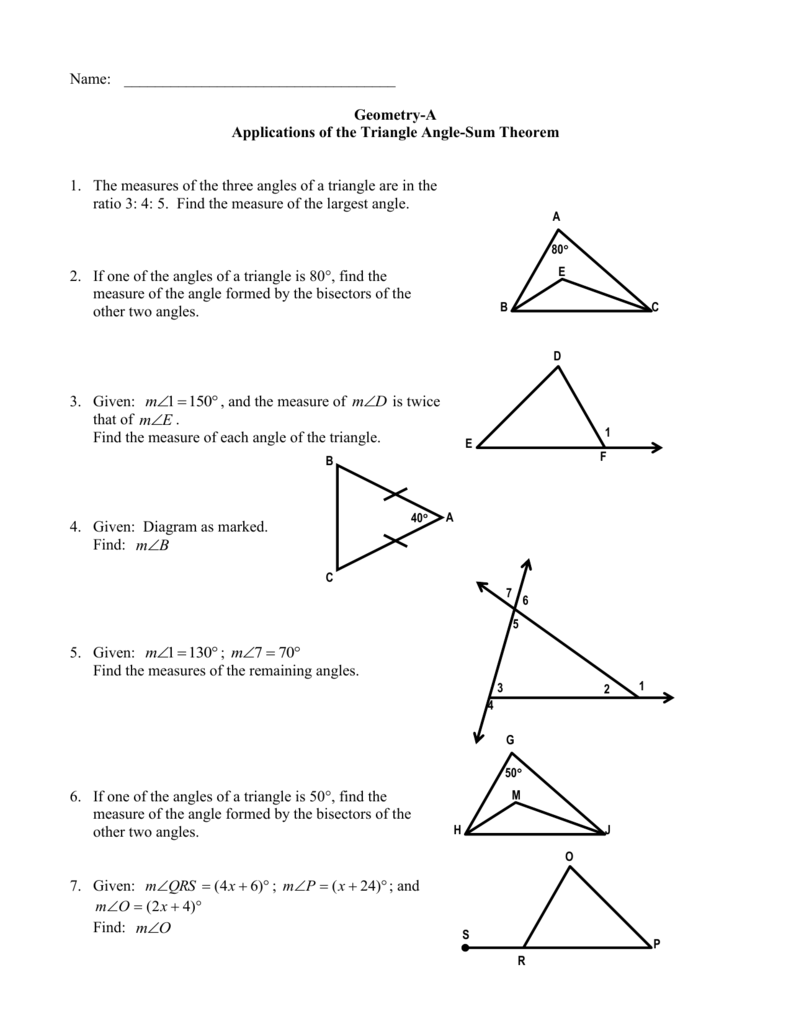
Applications Of The Triangle Angle
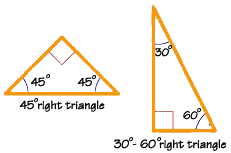
Like the 30°-60°-90° triangle, knowing one side length allows you to determine the lengths of the other sides.

Measurement 3 4 5 triangle angles. How does this right triangle calculator work?. And with the 3:4:5 triangle you can find your right angles without any complicated calculations. Thus the largest angle is 75°.
If you can "find" this triangle in your corner, you know the corner is square. Take angle O (not the 90 degree angle) c is the hypotenuse a is the side directly across from angle O b is the side adjacent to angle O SOH-CAH. Angle 3 is either angle B or angle A, whichever is NOT entered.
Additionally, the tool determined the last side length:. Hi on February 12, :. 4 5 6 - Acute scalene triangle, area=9.92.
The angles are in a ratio of 3:4:5. This is based on the Pythagorean Theorem from geometry:. It will even tell you if more than 1 triangle can be created.
They are equal to the ones we calculated manually:. You can use feet, meters, or any. The hypotenuse of the.
The measures of three angles of a triangle are in the ratio {eq}4:. Here’s the formula for the area of a triangle:. Find the angles of the triangle.
Any triangle with sides of 3, 4, and 5 feet will have a 90-degree angle opposite the 5-foot side. Note that the height forms a right angle with the base. Express in (i) Centesimal Measure, (ii) Radian Measure (take = 3.1415) Sol.
A Pythagorean triangle has measurements 3, 4 and 5. An acute triangle has 3 acute angles. Pick one leg of your project and measure out 3 feet from the corner.
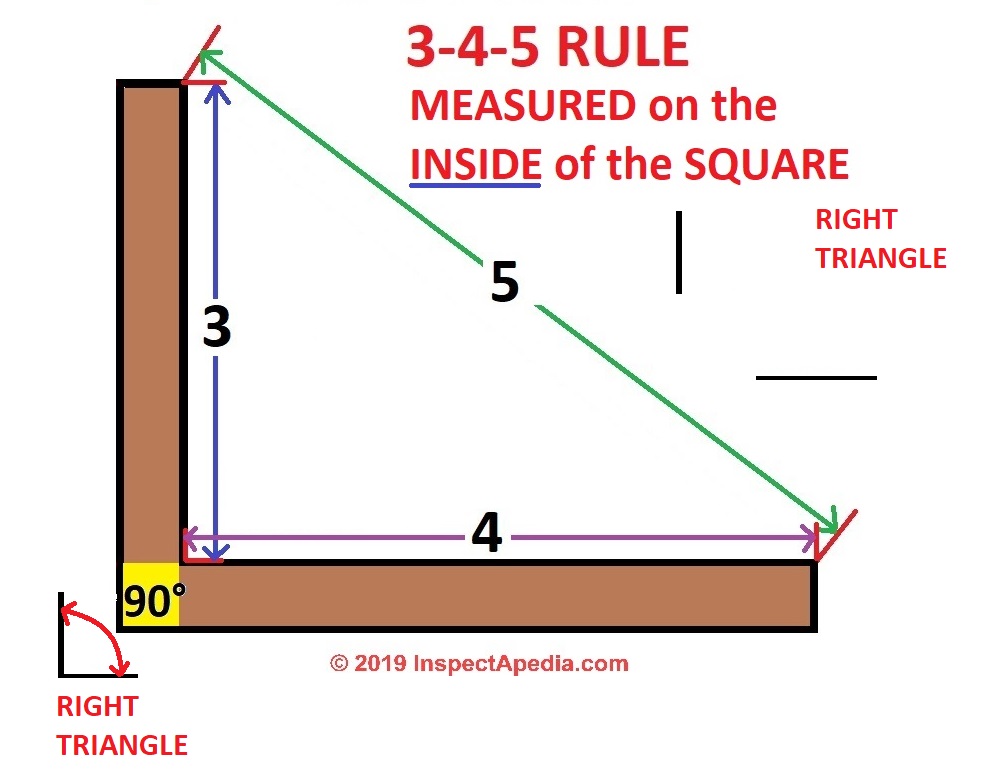
Relationship between measurement of the sides and angles in a triangle:. So, the three angles of a triangle are 30°, 60° and 90°. The beauty and simplicity of this technique are if the carpenter or builder needs to increase accuracy on larger walls or structures, any multiple of the 3-4-5 rule can be deployed.
To find the measure of the smallest angle of the triangle, we multiply 4 times 10. This figure shows a triangle with a base of 5 cm and a height of 2 cm:. Remember, the sum of the angles of a triangle is 180 degrees.
A = sin A/ sinB what is that formula. 123x = 180 Now, divide to isolate for x:. Angles In the triangle ABC, the ratio of angles is:.
Next, measure the length of the adjacent side to find the run. Create a right triangle. The other one is an isosceles triangle that has 2 angles measuring 45 degrees (45–45–90 triangle).
What is the distance of the center of gravity T from the vertex B?. Just take what you are given in a problem and try to determine what will make. Math Warehouse's popular online triangle calculator:.
⇒ x = 180/12. Sin(a) = 3/5 = 0.6. We know perimeter of a triangle is the sum of all its sides so According to problem we must have to find out the value of x° to be correct.
Express 1.2 radian in degree measure. The angles of a triangle (total of three angles), when added up. Triangles that do not have an angle measuring 90° are called oblique triangles.
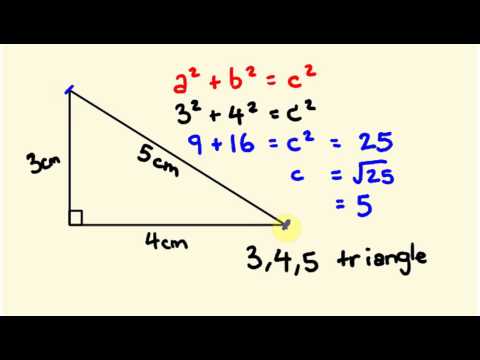
Of degrees in a triangle:. 3 2 + b 2 = 5 2 9 + b 2 = 25 b 2 = 16 => b = 4. Make a 3,4,5 Triangle !.
Put a mark on the board at the 3 feet point. Find the measure of each angle. Solve two triangle and 4 triangle in quadrilateral by use of sine rule.
To find the area of a triangle, you need to know the length of one side — the base (b for short) — and the height (h). I want to know the length of short side B. If a triangle has sides measuring 3, 4, and 5 feet (or any other unit), it must be a right triangle with a 90º angle between the short sides.
If the measures of the angles in a triangle are in the ration 3:4:5, the measure of the exterior angle of the triangle can not be a) 1D b)165D c)105D d)135D b 165 With the triangle ratio of 3:4:5 the equation can be made:. This is called an "angle-based" right triangle. P = 15 Semiperimeter:.
One of the two most famous is the 3–4–5 right triangle, where 32 + 42 = 52. 3 and 4 are the lengths of the shorter sides, and 5 is the length of the hypotenuse, the longest side. If one side is 3 units long and the other is 4 units, the hypotenuse will be 5 units if you have a true 90-degree angle between the sides.
Long side A is 3.6". 45 is the smallest angle in your triangle. I need to know how to get these and which way around they are.
A = 4 b = 5 c = 6 Area:. + B2 = C2 for a right triangle. To measure an acute angle without a protractor, start by drawing a vertical line connecting the 2 rays of the angle to form a right triangle.
A a triangle with angles of 30°, 60°, and 90° B an angle of 90° C a triangle with sides of 6, 8, and 10 D a triangle with sides of 3 and 4 E a triangle with a side measuring 4, next an angle of 90°, and next a side measuring 3 F a triangle with a side measuring 3, next an angle of 60°, and next a side measuring 4. Here the largest in ratio is 5 so we can write that 5x is the largest angle. The largest interior angle and side are opposite each other.
Well, we can create a virtual triangle between the endpoints by subtracting corresponding sides. The sum of the angles of a triangle is 180 degrees. Create an acute triangle.
The total number of degrees in the center is 360°. This simple ratio of 3:4:5 can be applied for the setting-out of any right-angle – all that is required is that the measurements used are in a ratio of 3:4:5, for example, a triangle with sides 600mm by 800mm by 1000mm, or. An obtuse triangle has 1 obtuse angle.
The angle of a triangle are in the ratio , find the smallest angle in degree and the greatest angle. The 45°-45°-90° triangle, also referred to as an isosceles right triangle, since it has two sides of equal lengths, is a right triangle in which the sides corresponding to the angles, 45°-45°-90°, follow a ratio of 1:1:√ 2. I know they are 90, 36.9 (to 1 dp) and 53.13 (to 2 dp).
This tool is designed to find the sides, angles, area and perimeter of any right triangle if you input any 3 fields (any 3 combination between sides and angles) of the 5 sides and angles available in the form. 3 23:47 Male / Under years old / Elementary school/ Junior high-school student / A little /. C = 17.78 in.
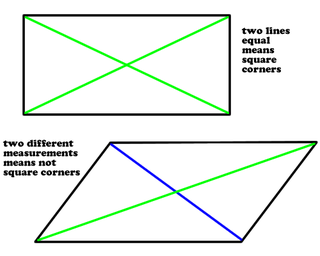
Use your tape measure to measure and mark one board at 3 feet and the other board at 4 feet. Their exterior angles are:. If the diagonal is 5 feet, then the triangle is a 3:4:5 right triangle and, by definition, the corner is square.
If a larger triangle is needed to increase accuracy of very large structures, any multiple of 3-4-5 could be used (such as a 6-8-10 foot triangle or a 9-12-15 foot triangle). So if the coordinates are (1,-6) and (4,8), the slope of the segment is (8 + 6)/(4 - 1) = 14/3. Sin(b) = 4/5 = 0.8.
A/sin A= b/sinB that i know. Angle C and angle 3 cannot be entered. For the best answers, search on this site https://shorturl.im/sQtGp.
And - you guessed it - one of the most popular Pythagorean triples is the 3-4-5 right triangle. A right-angled triangle with short sides of 3 units and 4 units will always have a longest side (hypotenuse) of 5 units. Medians 2:1 Median to side b (tb) in triangle ABC is 12 cm long.
Now, measure the adjacent board from the same corner to 4 feet and put a mark there. 3x + 4x + 5x = 180 x = 15 The angels of the triangle then are 45, 60, and 75. Angle L is a base angle and measures 72°.
A triangle is a geometrical object that has three angles, hence the name tri–angle.Any valid plane triangle must adhere to the following two rules:. A regular pentagon is created using the bases of five congruent isosceles triangles, joined at a common vertex. But the numbers 3,4,5 are easy to remember and no calculation is required.
135, 1 and 105 Thus choice b is not a correct responce. Sin, Cos and Tan equations. Angles of a Triangle.
In this situation, 3, 4, and 5 are a Pythagorean triple. Note that the angle formed by the adjacent side of the triangle and the opposite side measures 90 degrees. Create an obtuse triangle.
4 5 6 triangle. We know that, the sum of the three angles of a triangle = 180 ° 90 + (x + 1) + (2x + 5) = 180 ° 3x + 6 = 90 ° 3x = 84 ° x = 28 °. The angle c is 36°.
⇒ x = 15. This is shown as A squared + B squared = C squared and is known as the 3-4-5 rule in construction. B, Find the distance between T and the side b.
A 3-4-5 triangle is a right triangle. (1) the sum of two sides of a triangle must be greater than the third side, and (2) the sum of the angles of a plane triangle must be equal to 180°. We know the sum of angles of a triangle is equal to 180 degree.
Triangles by angle measure 4. From the theorem about sum of angles in a triangle, we calculate that γ = 180°- α - β = 180°- 30° - 51.06° = 98.94° The triangle angle calculator finds the missing angles in triangle. β = 51.06°, γ = 98.94°;.
According to the Pythagorean theorem, the sum of the squares of the two sides of a right-angled triangle equals the square of the hypotenuse. Angle 3 and Angle C fields are NOT user modifiable. First measure along one edge 3 feet.
C is the longest side (hypotenuse) and A and B are Step 2, Measure three units from the corner along one side. Angle (a) opposite 3:. The degree measures of the angles of a triangle are in the ratio 4 :.
A third side, connecting the two legs measuring 5 feet long;. A 3-4-5 triangle is right triangle whose lengths are in the ratio of 3:4:5. Solve problems with 3-4-5 right triangles.
Any triangle with sides of 3, 4 and 5 feet will have a 90 degree angle opposite the 5 foot side. You could of course use any dimensions you like, and then use Pythagoras' theorem to see if it is a right triangle. Enter any valid combination of sides/angles(3 sides, 2 sides and an angle or 2 angle and a 1 side) , and our calculator will do the rest!.
The answer is 40 degrees. Therefore, think of the ratios of 3:4:5 as variables followed by "x" (3x, 4x, and 5x). Computed angles, perimeter, medians, heights, centroid, inradius and other properties of this triangle.
How big are the angles a, b?. M<a ~ 36.87 degrees. Then, measure the distance between the two marks.
Angle measurements 3 4 5 triangle:. 3x + 4x + 5x = 180. Step 1, Understand the 3-4-5 method.
Angle (b) opposite 4:. Those triangles have side length requirements that aren't met by 3-4-5. If you allow the number of degrees in the first angles to be 3x, this means that the number of degrees in the second angle is equal to 4x and the number of degrees in the third angle is equal to 4x.
(180/12) = 15 , so x = 15 Now, substitute x for 15 in your variables:. If the measurement is less than 5 feet, the angle is too. I have a triangle with angles of 31, 59, and 90°.
When you are given the lengths of two sides of a right triangle, check the ratio of the lengths to see if it fits the 3:4:5 ratio. (ii) If the measure of an angle in degree and radian be D, G and C respectively, then. How to Use It.
Follow the directions of the answers above to find the angles. The answer is not 30-60-90. The measure along the adjacent edge 4 ft.
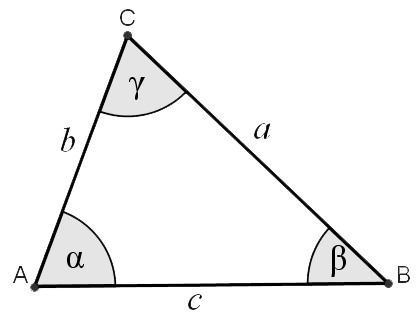
5, or of other special numbers such as the golden ratio. Using the law of sines makes it possible to find unknown angles and sides of a triangle given enough information. And you will have a right angle (90°).
The ratio of the length of a side of a triangle to the sine of its opposite angle is constant. The sum of the interior angles of a triangle will be 180°. In a right triangle, apart from the right angle, the other two angles are x + 1 and 2x + 5.
T = 9.92 2 Perimeter:. So, 4 x 10 = 40. An easy way to determine if the triangle is right, and you just know the coordinates, is to see if the slopes of any two lines multiply to equal -1.
For example, a right triangle may have angles that form simple relationships, such as 45°–45°–90°. You can put this solution on YOUR website!. ⇒ 12x = 180.
So let the ratio be x = 5x,4x and 3x 5x + 4x + 3x = 180 degree 12x = 180 degree. M<b ~ 53.13 degrees. Combine like terms and set the equation equal to 180, the amt.
A "side-based" right triangle is one in which the lengths of the sides form ratios of whole numbers, such as 3 :. If it is 5 feet, then you have a perfectly square corner. A right triangle has 1 right angle.
Therefore x° = 15. Triangle P2 Can triangle have two right angles?. No two angles can total to 180 degrees or more.
We do know that there is a 90 though, since 3-4-5 is a pythagorean triple. 5x = 5×15 = 75°. Again, this right triangle calculator works when you fill in 2 fields in the triangle angles, or the triangle sides.
Triangle with sides (4,3) blue Triangle with sides (8,5) pink What’s the distance from the tip of the blue triangle at coordinates (4,3) to the tip of the red triangle at coordinates (8,5)?.
Pythagorus Theorum Math Lesson 3 4 5 Triangle Youtube

The 3 4 5 Right Triangle Is A Commonly Used Right Triangle See Figure Use The Inverse Sine Function To Determine The Measure Of The Angle Opposite The Side Of Length 3 Express Your
Relations And Sizes Right Triangle Facts In Depth
Triangle Calculator

What Are The Angle Measurements Of A 3 4 5 Right Triangle Quora
Handy Tricks To Find Square 4 Steps Instructables
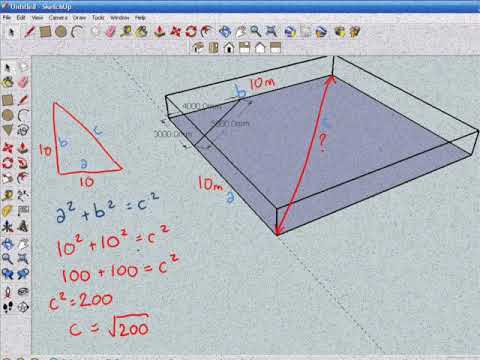
3 4 5 Rule Youtube
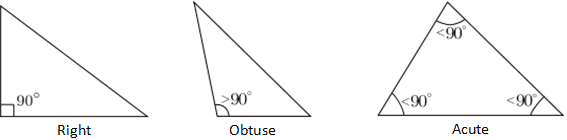
Triangle Calculator
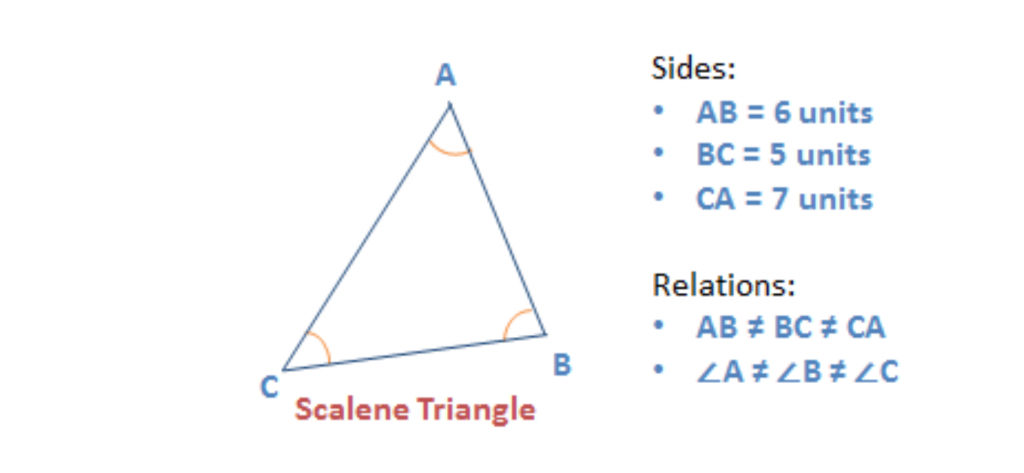
What Is A Triangle And Its Properties Definition Types Formulas

Right Triangle Angle And Side Calculator
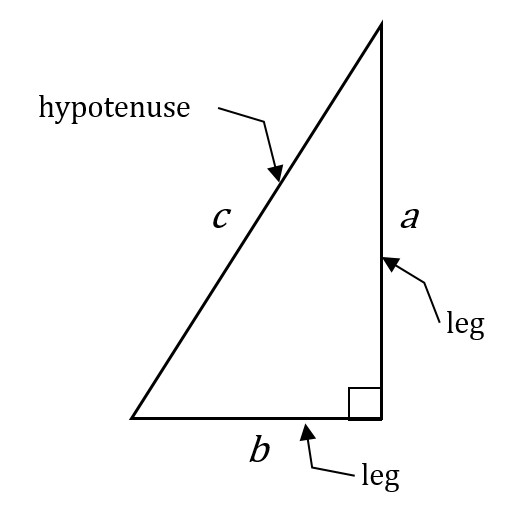
Right Triangles Gmat Free

Math Plane Did You Know

Getting Square With The 3 4 5 Triangle
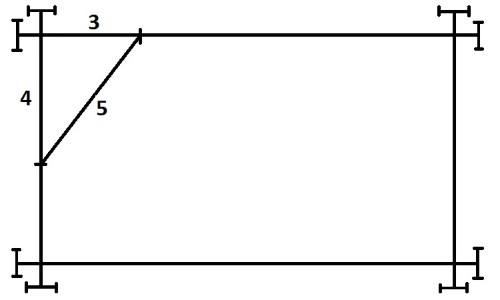
How To Square A Foundation For A Shed In 3 Simple Ways

Types Of Triangles Review Article Shapes Khan Academy
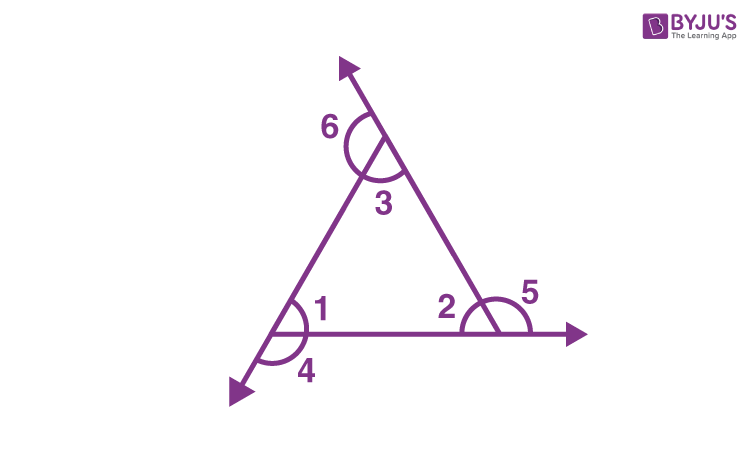
Triangles In Geometry Definition Types Properties Examples
The 3 4 5 Method For Squaring Corners Concord Carpenter

Triangles Ck 12 Foundation

Laying Out An Accurate Archery Range Using Stem Principles
Use Triangles Simple Geometry To Aid Building Framing
What Is Triangle Definition Facts Example

Properties Of 3 4 5 Triangles Definition And Uses Video Lesson Transcript Study Com
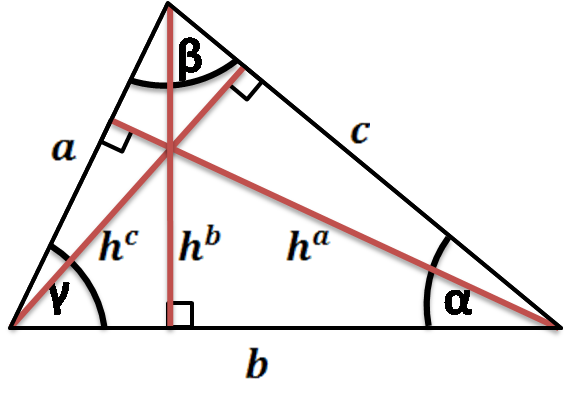
Height Of A Triangle Altitude Calculator Formulas

The Sides Of A Triangle Are In The Ratio 3 4 5 The Measure Of The Largest Angle Of The Triangle Brainly In
Www Brewtoncityschools Org Cms Lib Al Centricity Domain 133 5 5 The Triangle Inequality Pdf

Chapter 1 Basic Terms And Calculations

Cbse Ncert Solution For Class 6 Maths Understanding Elementary Shapes

Pythagorean Triangles And Triples
Pythagorean Theorem Wikipedia

How To Find The Length Of The Hypotenuse Of A Right Triangle Pythagorean Theorem Sat Math

G G 30 Interior And Exterior Angles Of Triangles 1 Investigate Justify
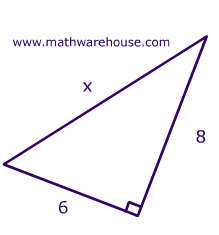
Right Triangles Hypotenuse Pythagorean Theorem Examples And Practice Problems
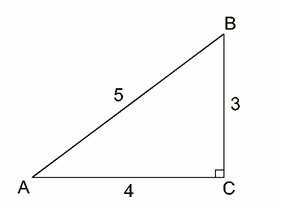
Math Scene Trigonometry Sine Cosine And Tangent Lesson 1
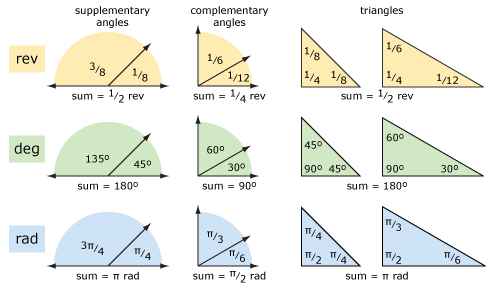
Angles The Revolution
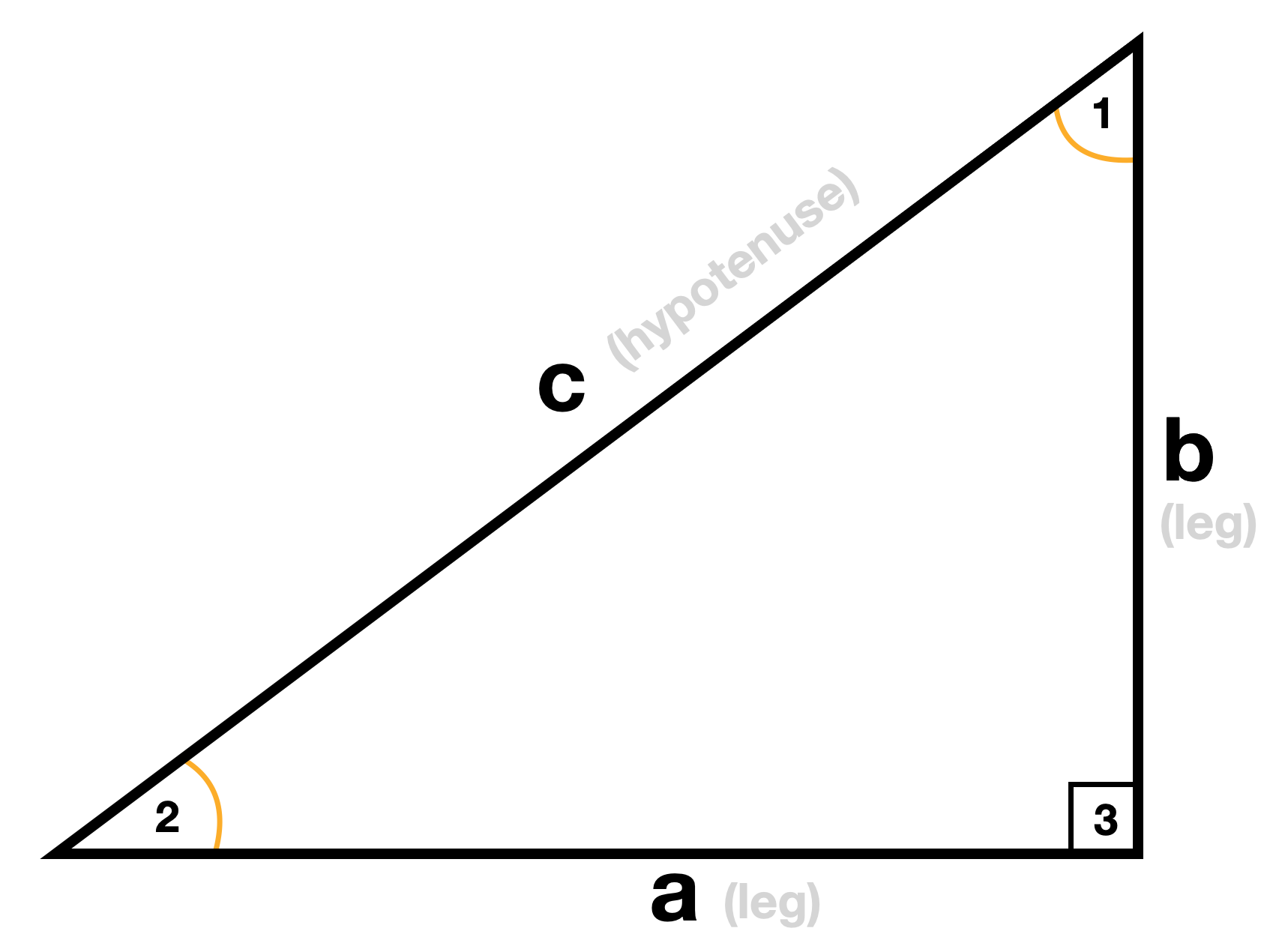
Right Triangle Calculator Pi Day

Interior And Exterior Angles Worksheets Questions And Revision Mme

The Ratio Of The Angles Of Triangle Abc Is 2 3 5 What Are The Angles Quora
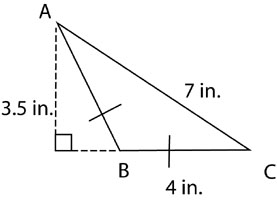
American Board
Q Tbn 3aand9gct 3otr1gbe3mn1ze0bfztuvnscogpwa Pavvijxx09x7ezvq3j Usqp Cau
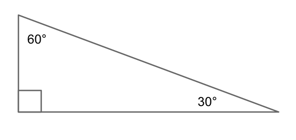
Triangles On Sat Math Geometry Strategies And Practice Problems

Angles Of A Triangle Free Math Help
Web Njit Edu Kappraff Activities Pdf

Triangle Calculator Square Footage Area
Solution The Measure Of The Angles Of A Triangle Are In The Ratio Of 4 5 6 Find The Measure Of The Largest Angle

Take Any Quadrilateral Say Abcd Fig 3 4 Divide It Into Two Triangles By Drawing A Diagonal You Get Six Angles 1 2 3 4 5 And 6 Use The Angle Sum Property Of
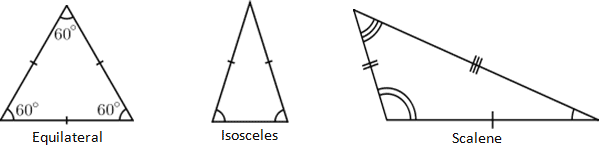
Triangle Calculator
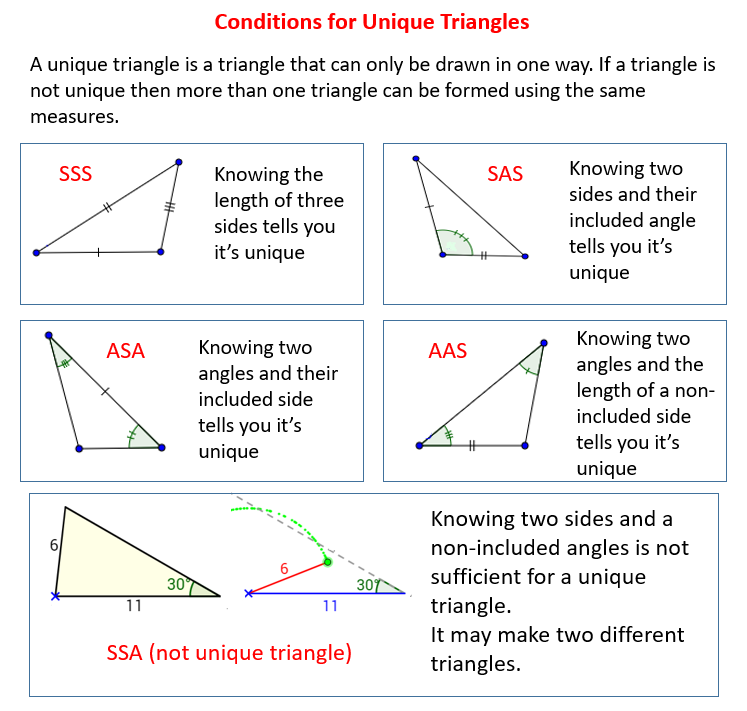
Conditions For A Unique Triangle Examples Solutions Videos Homework Worksheets Lesson Plans

Special Right Triangle Wikipedia
R D Sharma Solutions Class 9th Ch 9 Triangle And Its Angles Mcq S

3 4 5 Triangle
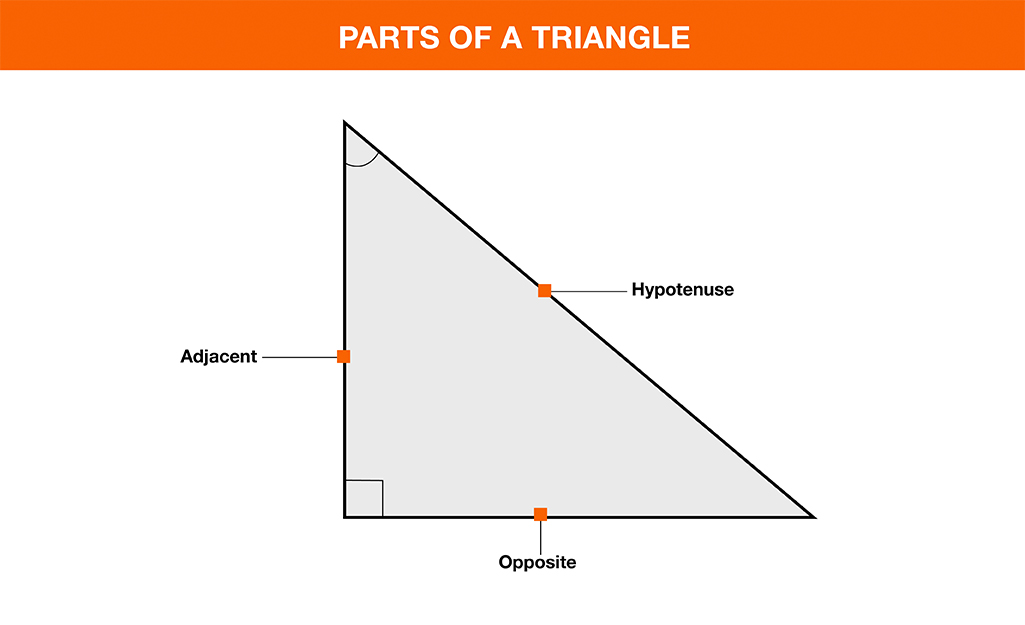
Tools To Measure Angles The Home Depot
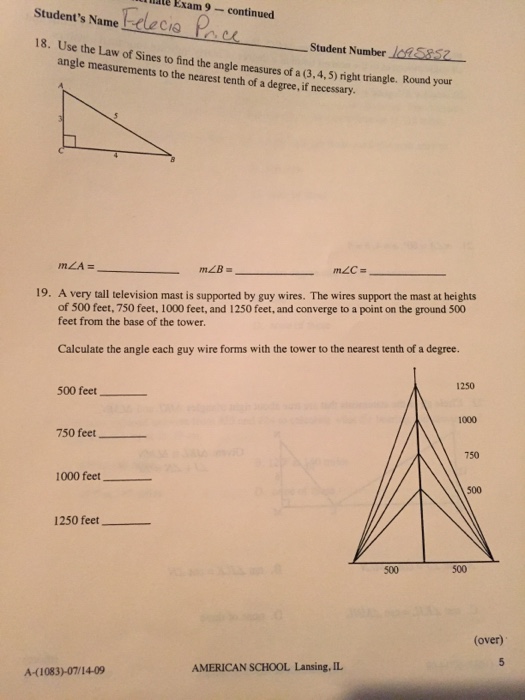
Solved Use The Law Of Sines To Find The Angle Measures Of Chegg Com

Pythagorus Theorum Math Lesson 3 4 5 Triangle Youtube
Q Tbn 3aand9gcs5rv5kynczivnjbnvistxlh 9 Ulikvhauqvlz3b5wqlgsrb4x Usqp Cau

Unit 5 Section 6 Finding Angles In Triangles

3 4 5 Triangle Angles Sides How To Solve Full Lesson

The Sides Of A Triangle Are In The Ratio 3 4 5 If It S Perimeter Is 36 Cm Area Of Triangle Is Brainly In
Q Tbn 3aand9gcsgbkagqtextbltqgjp8btolnoivup 3mujb2ky9pwca39e4ka1 Usqp Cau

Mathematics Information Triangle Poster Measurement Mathematics Blue Angle Png Pngegg
Math Scene Trigonometry Sine Cosine And Tangent Lesson 1
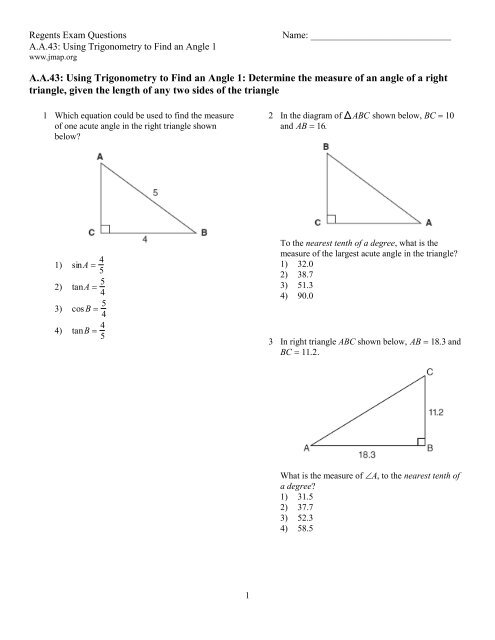
A A 43 Using Trigonometry To Find An Angle 1 Determine The

Rd Sharma Solutions For Class 10 Chapter 5 Trigonometric Ratios Exercise 5 1 Get Pdf For Free

3 4 5 Triangle Definition Math Open Reference
R D Sharma Solutions Class 9th Ch 9 Triangle And Its Angles Mcq S

Ratios In Right Triangles Ppt Video Online Download
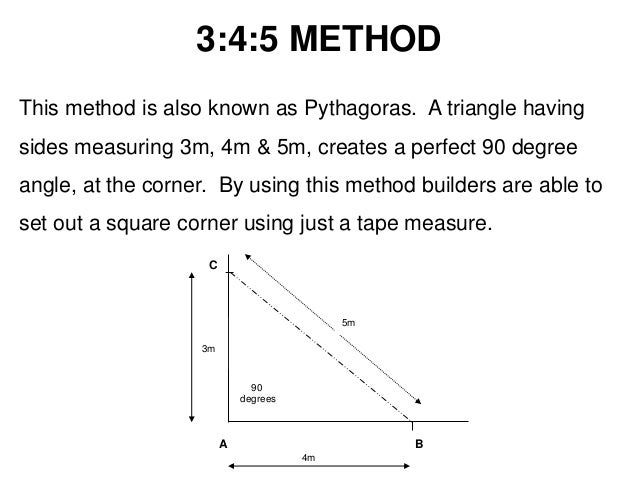
Tutor L1 Setting Out

5 12 13 Triangle Angles Sides How To Solve Full Lesson
Of Sidesname Of Diagonalsinterior Angle Sum Exterior Angle Sum Each Interior Angle If Regular Each Exterior Angle If Regular Ppt Download

Right Triangle Calculator Definition Formula

3 4 5 Right Triangles Explanation Examples

Pythagoras Theorem Triangles And Trigonometry Mathigon
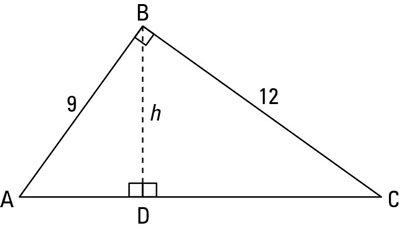
How To Solve Problems With The Altitude 0n Hypotenuse Theorem Dummies

7 Inch Carpenter Speed Square Triangle R Buy Online In Cambodia At Desertcart

Triangle Wikipedia

Classifying Triangles Ck 12 Foundation
Www Lcps Org Cms Lib4 Va Centricity Domain 3279 Practice proving triangles similarkey Pdf

3 4 5 Triangle Angles Sides How To Solve Full Lesson

Angles Of A Triangle Free Math Help
Ex 11 1 7 Draw A Right Triangle Where Sides Other Than Hypotenuse
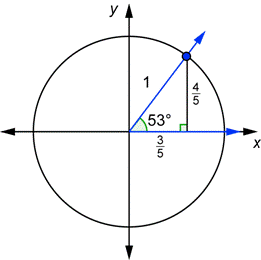
Unit Circle Trigonometry

3 4 5 Triangle Definition Math Open Reference
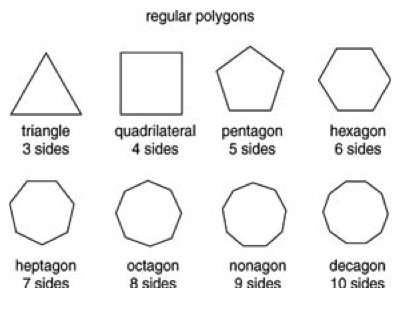
How Do You Find The Measure Of Each Interior Angle Of A Polygon Socratic

Times Module M15 Pythagoras Theorem
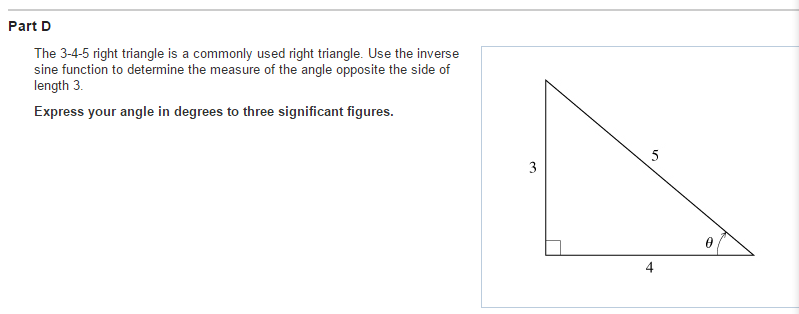
Solved Part Dthe 3 4 5 Right Triangle Is A Commonly Used Chegg Com
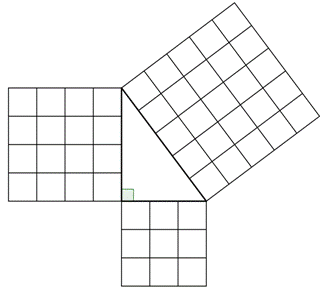
The Pythagorean Theorem
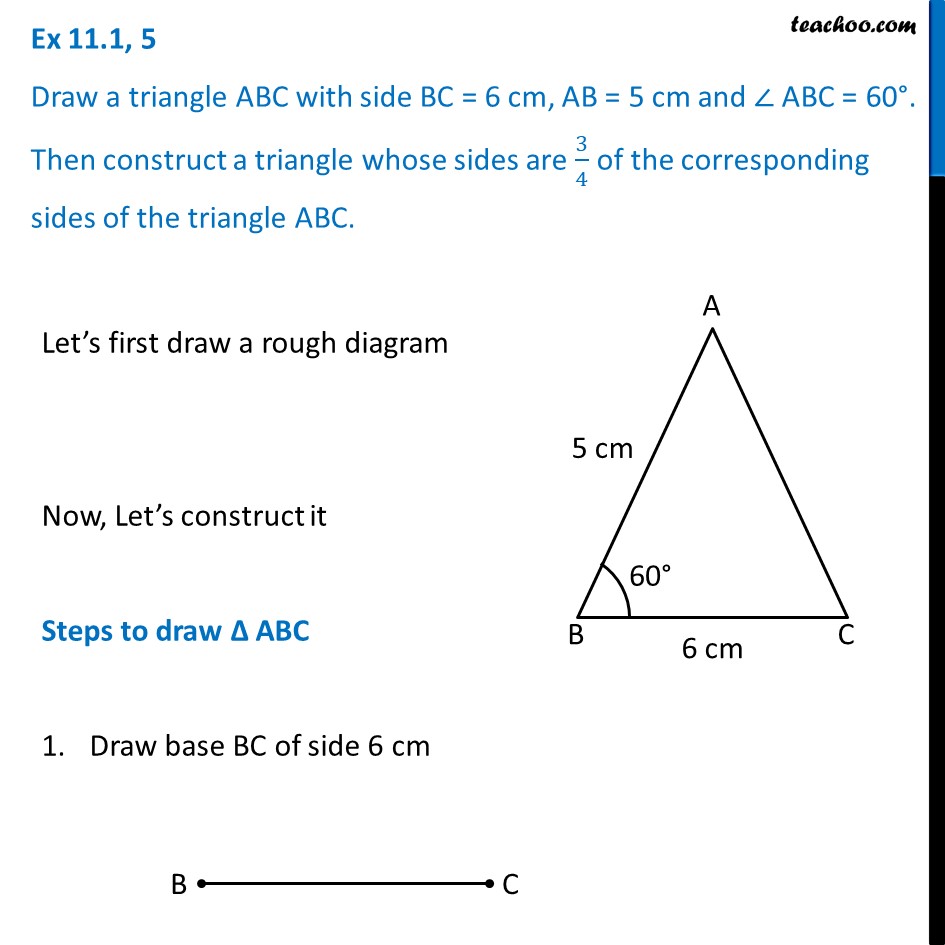
Ex 11 1 5 Draw A Triangle Abc With Side 6 Cm Ab 5 Cm Angle
Q Tbn 3aand9gcs Rk4lbq2y 17q6nwyvcb0pf1dneyfvrufn6g0uvskpz5wk 4n Usqp Cau
Pythagorean Triangles And Triples
2

Unit 3 Section 5 Constructions And Angles
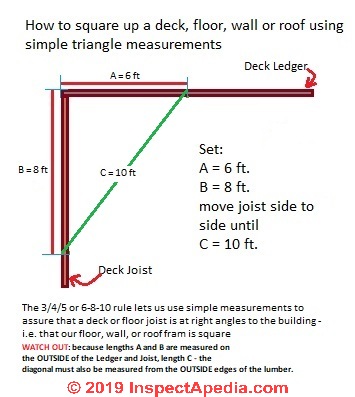
Use Triangles Simple Geometry To Aid Building Framing
Solution If There Is A Triangle The Measurements Of The Three Sides Are 6 8 And 10 What Is The Altitude Of The Triangle It Says To Write A Proportion And Solve
Www Brewtoncityschools Org Cms Lib Al Centricity Domain 133 5 5 The Triangle Inequality Pdf
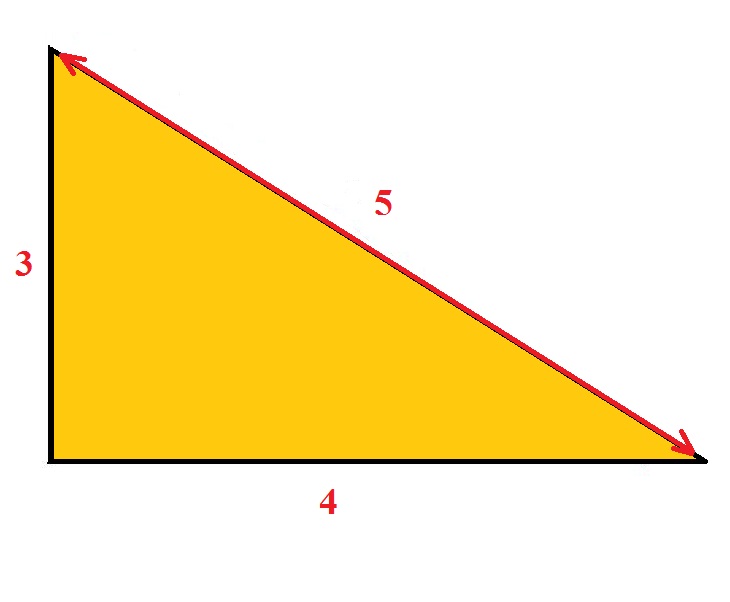
How To Check For Square
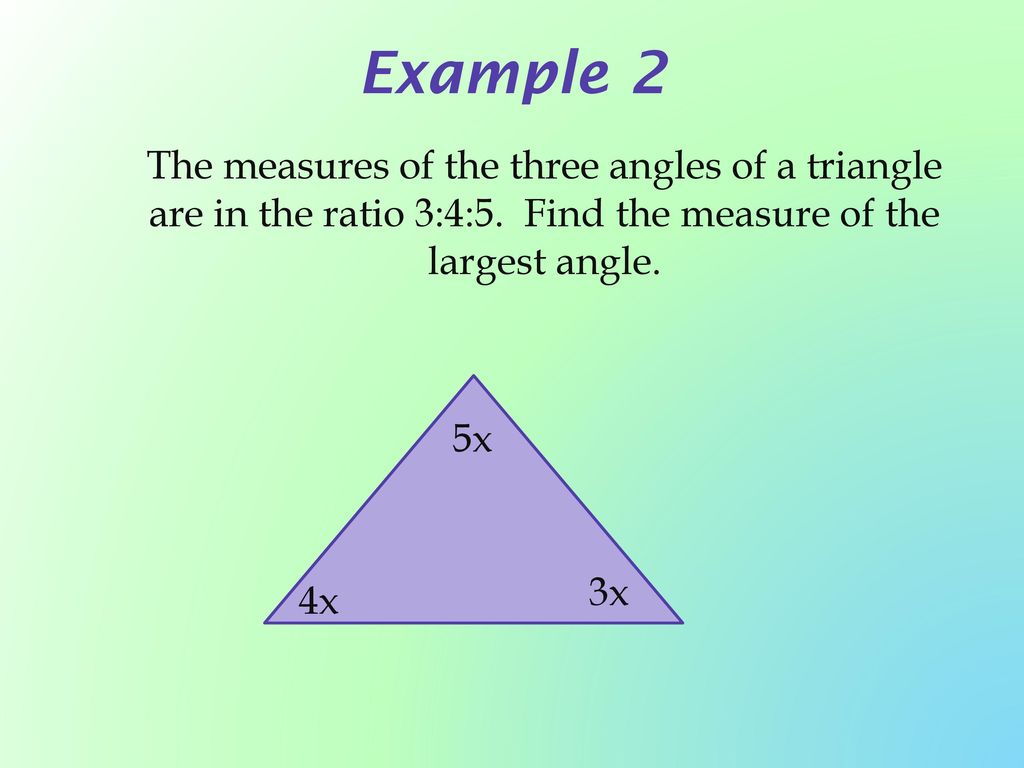
7 1 Triangle Application Theorems Ppt Download

4 Setting Out Right Angles And Perpendicular Lines
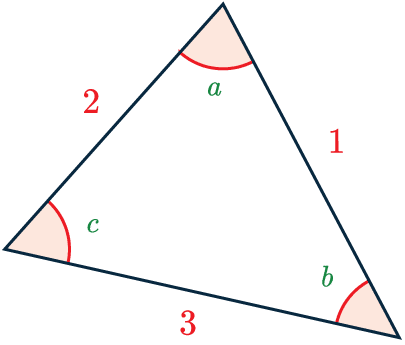
Interior And Exterior Angles Worksheets Questions And Revision Mme

How To Use The 3 4 5 Rule To Build Square Corners 4 Steps



